3-7; Sinusoidal Functions

Chapter 3, Lesson 7: Sinusoidal Functions as Mathematical Models

Main Objective: "To write an equation using sine or cosine functions and use the equation as a matematical model to make predictions and interpretations about the real world"

Using what we have learned in past chapters:

y=C + A(Sin or cos) (B(x or theta - D))

*use "X" when in radian mode
*use "theta" when in degree mode

C= sinusoidal axis (vertical translation)
A= amplitude (vertical dilation)
B= 360/period (horizontal dilation)
D= phase displacement (horizontal translation)

Radians converted to degrees: 180/pi
Degrees converted to radians: pi/180

Tip: After looking at the word problem decide if you should use sine or cosine.

Use sine: when you are dealing with the location of the midpoint of a graph
Use cosine: when you are dealing with the maximum of a graph

Tip: After looking at the word problem decide if you should use radians or degrees:

*Use radians: when you want to eliminate the confusion of having different units

Summary:

This lesson basically combines the things we have learned about sin and cos functions with what we have learned about radians and degrees and puts them in a real world situation, using circular graphs to depict situations that we need to translate into sinusoidal functions.

There are 2 graphs you might have to use in word problems:

A circle with P= point on outer rim, r= radius, d= distance to ground (ex. wheel problems) and the sinusoid graphs (sin and cos) we have learned about

Example:

Suppose that the waterwheel in the figure rotates at 6 revolutions per minute. Two seconds after you start a stopwatch, point P on the rim of the wheel is at its greatest height, d=13 ft, above the surface of the water. The center of the waterwheel is 6ft above the surface.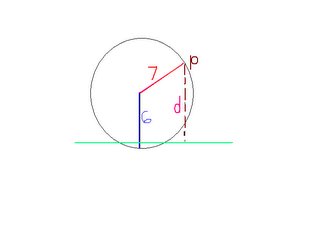

a. Sketch the graph of d as a function of t since you started the stopwatch.

b. Assuming that d is a sinusoidal function of t, write a particular equation.

c. How high above or below the water's surface will P be at time t=17.5 sec?

d. At what time (t) was point P first emerging from the water?

Answer:

a. From the information we are given, you can tell that the sinusoidal axis is located at d=6 (this is the center of the waterwheel). Because you know the sinusoidal axis you can figure out the high and low points by adding and subtracting the amplitude (7).

6+7= 13 (high point)

6-7= -1 (low point)

Sketch the high point at t=2 because point P is at its maximum after 2 seconds

Because the waterwheel rotates at 6 revolutions per minute, the period is 60/6= 10 seconds, or 1 revolution in 10 seconds. This means you would mark the next high point at t=2 + 10=12

*Continue by marking a low point halfway between the two high points, and mark the points of inflection on the sinusoidal axis halfway between each consecutive high and low*

The graph should look like the following:

b. Remember: d=C+AcosB(t-D) As we can see from the graph, C=6, A=7, and D=2

Because the period of this sinusoid is 10, using radians the "B" would be calculated as 10/2(pi), which equals 5/(pi)

*Don't forget*- B is the RECIPROCAL of the horizontal dilation, therefore B= pi/5

Using the formula above you would write the answer as d=6+7cos((pi/5)(t-2))

c. Set the window on your grapher to include 17.5. Then trace or scroll to this point. By looking at the graph you can determine that d= -.6573 and is going up.

d. Point P is either submerging into or emerging from the water when d=0. At the first zero the point is going into the water. At the next zero, the point is emerging. Using intersect or zeros on your calculator you will find points at:

t= 7.8611...rounded to 7.9 seconds

For Additional Problems or help with Sinusoids in general (which can help you with sinusoidal model problems) go to http://www.nsa.gov/teachers/hs/trig16.pdf

This is a picture of my puppy Luna, who I'm raising to be a Guide Dog.

Landry, you are next! Good Luck!